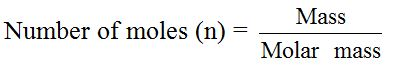TOPIC 4: THE MOLE CONCEPT AND RELATED CALCULATIONS
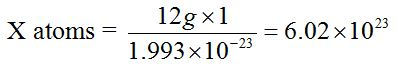
The Mole as a Unit of Measurement
The Mole with Other Units of Measurements
Compare the mole with other units of measurements
When carrying out an experiment, a chemist cannot weigh out a single atom, ion, electron, proton or molecule of a substance. These particles are simply very small. A counting unit that is useful in practical chemistry must be used.
The standard unit is called one mole of the substance. One mole of each of these different substances contains the same number of the particles (atoms, molecules, ions, electrons, protons, neutrons, etc). That number per mole has been worked by several different experimental methods and is found to be 6.0 × 1023. The value 6.0 × 1023 is called Avogadro’s constant or Avogadro’s number and is abbreviated as L. It is named after the nineteenth-century Italian chemist, Amedeo Avogadro.
The value 6.0 × 1023 is obtained through the following relationship.The mass of one atom of carbon-12 is 1.993 × 10-23g. Then, the number of atoms present in 12g of carbon-12 is derived as follows:
1 atom = 1.993 × 10-23g
X atoms = 12g
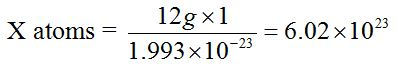
X = 6.0 × 1023 atoms.
Therefore, the number of atoms in 12g of carbon-12 and hence the number of particles in a mole are 6.02 × 1023 atoms.
Hence, Avogadro’s number is the number of atoms in exactly 12g of carbon-12 isotope.One mole of any substance contains as many as many elementary particles as the Avogadro’s number (constant).
So, from the above explanation, the mole can be defined as the amount of a substance that contains as many elementary particles as the number of atoms present in 12g of carbon-12 isotope.
| Substance | Formula | Relative formula mass, Mr | Mass of one mole (molar mass) | This mass (1 mole) contains |
| Carbon | C | 12 | 12g | 6.0 × 1023 carbon atoms |
| Iron | Fe | 56 | 56g | 6.0 × 1023 iron atoms |
| Hydrogen | H2 | 2 × 1 = 2 | 2g | 6.0 × 1023 molecules |
| Oxygen | O2 | 2 × 16 = 32 | 32 | 6.0 × 1023 molecules |
| Water | H2O | (2×1) + 16 = 18 | 18g | 6.0 × 1023 formula units |
| Magnesium oxide | MgO | 24 + 16 = 40 | 40g | 6.0 × 1023 formula units |
| Calcium carbonate | CaCO3 | 40+12+(3×16) = 100 | 100g | 6.0 × 1023 formula units |
| Silicon oxide | SiO2 | 28 + (2 × 16) = 60 | 60g | 6.0 × 1023 formula units |
| Fe3+ | Fe3+ | 56 | 56g | 6.0 × 1023 iron(III) ions |
| Cl– | Cl– | 35.5 | 35.5g | 6.0 × 1023 chloride ions |
| e– | e– | – | – | 6.0 × 1023 electrons |
The other substances, which also exist as molecules, include ozone molecule (gas), O3; phosphorus molecule (solid), P4; sulphur molecule, S8, etc.
In real life, when dealing with large numbers of small objects, it is usual to count them in groups. The objects are grouped and counted in unit amounts. For example, we buy a carton of soap, a gallon of kerosene, a crate of soda, a dozen of pencils, a ream of papers, etc.
Some units of measurement
| Unit | Number of objects per unit |
| Pair | 1 pair = 2 objects, e.g. gloves, shoes, socks, scissors, etc are always sold in pairs. |
| Dozen | 1 dozen = 12 objects e.g. a dozen of cups, plates, spoons, etc. |
| Gross | 1 gross = 144 objects, e.g. a box of blackboard chalk contains 144 pieces of chalk. |
| Ream | 1 ream = 500 objects, e.g. papers are sold in reams of 500 sheets. |
| Mole | 1 mole = 6.02 ×1023 particles. In chemistry, extremely small particles are expressed in moles. For example:1 mole of atoms = 6.02 ×1023 atoms1 mole of electrons = 6.02 ×1023 electrons1 mole of protons = 6.02 ×1023 protons1 mole of ions = 6.02 ×1023 ions1 mole of molecules = 6.02 ×1023 molecules |
Molar Quantities of Different Substances
Measure molar quantities of different substances
The mass of one mole of any substance (its molecular mass) is the atomic mass or molecular mass expressed in grams (or kilograms). For convenience, chemists prefer to express mass in grams, although the SI unit of mass is the kilogram. This is because the amount of substances which chemists usually work with in science laboratories, is quite small and if their masses are expressed in kilograms, the numbers used would be extremely small.
You can calculate the molar mass (M) of any substance by summing up the relative atomic weights of its constituents atoms. For example, ethanol, C2H5OH, contains two carbon atoms, six hydrogen atoms and one oxygen atom. So, the molar mass of ethanol can be calculated thus: Molar mass of C2H5OH = (2 × 12) + (6×1) + 16 = 46g.
In a similar way, molar masses of other compounds can be calculated. For example, the molar mass of sodium chloride, NaCl, is calculated by adding together the relative atomic masses of the constituents elements (Na = 23 and Cl = 35.5) = 23 + 35 = 58.5g (g mol-1).
It is important to note that relative atomic mass or relative molecular mass has no unit while molar masses are always expressed in grams or kilograms.
The molar mass of a compound is the same as the relative molecular mass and the molar mass of an element is the same as the relative atomic mass (Ar) of that element. The only difference lies in the units.
Example 1
- M(CO2) = 44g (or g mol-1) = molar mass of carbon dioxide
- Mr(CO2) = 44 = relative molecular mass of carbon dioxide
- M(Fe) = 56g (or g mol-1) molar mass of iron
- Mr(Fe) = 56 = Relative atomic mass of iron
Similarly, the molar masses of each of the following substances can be calculated using values for the relative atomic masses of the elements.
Molar masses of different substances
| Substance | Formula | Molar mass |
| Ammonia | NH3 | 14 + 1×3 = 17g |
| Ammonium chloride | NH4Cl | 14 + (1×4) + 35.5 = 53.5g |
| Lead (II) nitrate | Pb(NO3)2 | 207 + (14×2) + (16×6) = 331g |
| Sulphuric acid | H2SO4 | (1×2) + 32 + (16×4) = 98g |
| Calcium carbonate | CaCO3 | 40 + 12 + (16×3) = 100g |
| Potassium dichromate | K2Cr2O7 | (39×2) + (52 ×2) + (16×7) = 294g |
Application of the Mole Concept